Years
>
Year 11>
Sequences and Series>
Sum of Infinite Geometric Series>
Sum of Infinite Geometric SeriesSum of Infinite Geometric Series
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 8 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- Answered
- Review
-
Question 1 of 8
1. Question
A kangaroo slows down its jumping as it approaches a lake.
Starting at 66m, its next jump covers half of the previous jump. Find the limiting sum of the distances that the kangaroo jumps over.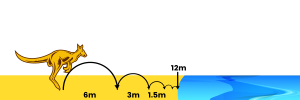
- S∞=S∞= (12)mm
Hint
Help VideoCorrect
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Limiting Sum Formula
S∞=a1−rS∞=a1−rwhere -1where −1<<rr<<11Common Ratio Formula
r=U2U1=U3U2r=U2U1=U3U2First, solve for the value of rr.rr == U2U1U2U1 == 3636 Substitute the first and second term == 1212 Simplify Next, substitute the known values to the formulaFirst termFirst term[a][a] == 66 Common RatioCommon Ratio[r][r] == 1212 S∞S∞ == a1−ra1−r S∞S∞ == 61−1261−12 Substitute known values == 612612 Evaluate == 1212 S∞=12 mS∞=12 m -
Question 2 of 8
2. Question
Find the limiting sum1-13+19-127…1−13+19−127…Write fractions as “a/b”- S∞=S∞= (3/4)
Hint
Help VideoCorrect
Excellent!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Limiting Sum Formula
S∞=a1−rS∞=a1−rwhere -1where −1<<rr<<11Common Ratio Formula
r=U2U1=U3U2r=U2U1=U3U2First, solve for the value of rr.rr == U2U1U2U1 == −131−131 Substitute the first and second term == -13−13 Next, substitute the known values to the formulaFirst termFirst term[a][a] == 11 Common RatioCommon Ratio[r][r] == -13−13 S∞S∞ == a1−ra1−r S∞S∞ == 11−(−13)11−(−13) Substitute known values == 143143 Evaluate == 3434 S∞=34S∞=34 -
Question 3 of 8
3. Question
Find the value of rr given that:S∞=18S∞=18U1=6U1=6Write fractions as “a/b”- r=r= (2/3)
Hint
Help VideoCorrect
Nice Job!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Limiting Sum Formula
S∞=a1−rS∞=a1−rwhere -1where −1<<rr<<11Substitute the known values then solve for rrS∞S∞ == 1818 1st term1st term == 66 S∞S∞ == a1−ra1−r 1818 == 61−r61−r Substitute known values 18(1-r)18(1−r) == 66 Cross multiply 18-18r18−18r == 66 Distribute 18-18r18−18r -18−18 == 66 -18−18 Subtract 1818 from both sides -18r−18r ÷(-18)÷(−18) == -12−12 ÷(-18)÷(−18) Divide both sides by -18−18 rr == 12181218 rr == 2323 Simplify r=23r=23 -
Question 4 of 8
4. Question
Given that S∞=45S∞=45, find the value of xxx+x2+x3…x+x2+x3…Write fractions as “a/b”- x=x= (4/9)
Hint
Help VideoCorrect
Well Done!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Limiting Sum Formula
S∞=a1−rS∞=a1−rwhere -1where −1<<rr<<11Common Ratio Formula
r=U2U1=U3U2r=U2U1=U3U2First, solve for the value of rr.rr == U2U1U2U1 == x2xx2x Substitute the first and second term == xx Next, substitute the known values then solve for xxS∞S∞ == 4545 First termFirst term[a][a] == xx Common RatioCommon Ratio[r][r] == xx S∞S∞ == a1−ra1−r 4545 == x1−xx1−x Substitute known values 4(1-x)4(1−x) == 5x5x Cross multiply 4-4x4−4x == 5x5x Distribute 4-4x4−4x +4x+4x == 5x5x +4x+4x Add 4x4x to both sides 44÷9÷9 == 9x9x÷9÷9 Divide both sides by 99 4949 == xx xx == 4949 x=49x=49 -
Question 5 of 8
5. Question
An acrobatic stunt plane flies vertically upwards. The further it goes up, the more it allows gravity to take over its flight, slowing it down until it reaches a peak point. At the first second, it travels 120120m, 4040m at the next, then 13131313m, and so on. Find the total distance that the plane travels upwards until reaching the peak point.- S∞=S∞= (180)mm
Hint
Help VideoCorrect
Correct!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Limiting Sum Formula
S∞=a1−rS∞=a1−rwhere -1where −1<<rr<<11Common Ratio Formula
r=U2U1=U3U2r=U2U1=U3U2First, solve for the value of rr.rr == U2U1U2U1 == 4012040120 Substitute the first and second term == 1313 Simplify Next, substitute the known values to the formulaFirst termFirst term[a][a] == 120120 Common RatioCommon Ratio[r][r] == 1313 S∞S∞ == a1−ra1−r S∞S∞ == 1201−131201−13 Substitute known values == 1202312023 Evaluate == 180180 S∞=180 mS∞=180 m -
Question 6 of 8
6. Question
A ball was dropped at a height of 10 m10 m. Find the limiting sum if the ball bounces back up by 4/54/5 of that height.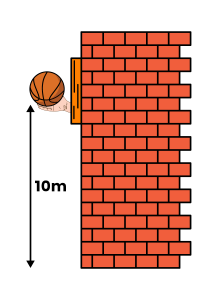
- S∞=S∞= (90)mm
Hint
Help VideoCorrect
Keep Going!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Limiting Sum Formula
S∞=a1−rS∞=a1−rwhere -1where −1<<rr<<11First, find the value of the first term (aa).The first term would be the first time the ball bounces, which is 4/5 of 10.10×45 = 8 Substitute the known values to the formulaNote that the ball will be bouncing up and down each time, so the value must be multiplied by 2.r = 45 a = 8 S∞ = a1−r = 2(81−45) Substitute known values = 2(815) Evaluate = 2(40) = 80 Finally, add the height from where the ball was first dropped, which is 10.S∞ = 80 +10 = 90 S∞=90 m -
Question 7 of 8
7. Question
A speeding car brakes and travels 18 m in the first second, 4.5 m in the second, and 1.125 m in the third. How far will the car travel until it completely stops?
- S∞= (24)m
Hint
Help VideoCorrect
Fantastic!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Limiting Sum Formula
S∞=a1−rwhere -1<r<1Common Ratio Formula
r=U2U1=U3U2First, solve for the value of r.r = U2U1 = 4.518 Substitute the first and second term = 14 Simplify Next, substitute the known values to the formulaFirst term[a] = 18 Common Ratio[r] = 14 S∞ = a1−r S∞ = 181−14 Substitute known values = 1834 Evaluate = 24 S∞=24 m -
Question 8 of 8
8. Question
A man is standing on the roof of a building and drops a ball which travels 36m onto the ground below. As it touches the ground, it goes back up 23 of its previous height and continues to dribble. Find out how high the ball rises after its sixth bounce.(i) How high would the ball rise after its sixth bounce?(ii) How many bounces will it take for the ball to have a height of 719m?(iii) Find the total sum of the heights of the ball until it stopsRound off answer to 2 decimal places when applicable-
(i) (3.16)m(ii) (4) bounces(iii) (180)m
Hint
Help VideoCorrect
Excellent!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Common Ratio Formula
r=U2U1=U3U2Limiting Sum Formula
S∞=a1−rwhere -1<r<1General Rule of a Geometric Sequence
Un=arn−1(i) Find how high the ball would rise after its sixth bounce.First, sketch the first few data on how the ball bounces.Note that each succeeding height is computed by getting 23 of the previous heightNext, substitute the known values to the general ruleNumber of terms[n] = 6 First term[a] = 24 Common Ratio[r] = 23 Un = arn−1 U6 = 24⋅236−1 Substitute known values = 24⋅235 Evaluate = 24⋅32243 = 768243 = 3.16m The height of the ball after the sixth bounce is 3.16m(ii) Find how many bounces it will take for the ball to have a height of 719m.Substitute the known values to the general rulenth term[Un] = 719 First term[a] = 24 Common Ratio[r] = 23 Un = arn−1 719 = 24⋅23n−1 Substitute known values 649⋅124 = 24⋅23n−1⋅124 Multiply both sides by 124 827 = 23n−1 log827 = log23n−1 Take the logarithm of both sides log827 = (n−1)log23 log827log23 = n−1 1+log827log23 = n 1+3 = n n = 4 It takes 4 bounces for the ball to have a height of 719m(iii) Find the total sum of the heights of the ball until it stops.First, substitute the known values to the limiting sum formulaFirst term[a] = 24 Common Ratio[r] = 23 S∞ = a1−r S∞ = 241−23 Substitute known values = 2413 Evaluate = 72 This is just the total of the distances traveled by the ball from the air to the ground.It does not include the distance when it bounces from the ground back to the air and the initial height of 36mFinally, double the limiting sum and add 362(72)+36 = 144+36 = 180m The total sum of the heights of the ball until it stops is 180m(i) 3.16m(ii) 4 bounces(iii) 180m -





