Years
>
Year 10>
Fractions>
Fraction Word Problems: Multiplication and Division>
Fraction Word Problems: Multiplication and DivisionFraction Word Problems: Multiplication and Division
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 6 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- 4
- 5
- 6
- Answered
- Review
-
Question 1 of 6
1. Question
How many `1 1 / 6` sections of rope are there in a total length of `42`m?- (36)
Hint
Help VideoCorrect
Correct!
Incorrect
Transforming a Fraction from Mixed to Improper
`=` $$\frac{(\color{#9a00c7}{c}\times\color{#00880A}{A})+\color{#007DDC}{b}}{\color{#9a00c7}{c}}$$ First, list down the values stated in the problemTotal length of the rope `=` `42`m Sections it will be cut into `=` $$\color{#00880A}{1}\frac{\color{#007DDC}{1}}{\color{#9a00c7}{6}}=\frac{(\color{#9a00c7}{6}\times\color{#00880A}{1})+\color{#007DDC}{1}}{\color{#9a00c7}{6}}=\frac{6+1}{6}=\frac{7}{6}$$ Divide the two values`42-:7/6` `=` `42/1xx6/7` Reciprocate the divisor and change the operation to multiplication `=` `(7xx6)/1xx6/(7xx1)` `42` and `7` are both multiples of `7` `=` $$\frac{\color{#CC0000}{7}\times6}{1}\times\frac{6}{\color{#CC0000}{7}\times1}$$ Cancel diagonally `=` `6/1xx6/1` `=` `36/1` `=` `36` `36` -
Question 2 of 6
2. Question
A race track is `3 2/3`km long per lap. How many laps will a racer need to make in order to complete a `77`km race?- (21) laps
Hint
Help VideoCorrect
Keep Going!
Incorrect
Transforming a Fraction from Mixed to Improper
`=` $$\frac{(\color{#9a00c7}{c}\times\color{#00880A}{A})+\color{#007DDC}{b}}{\color{#9a00c7}{c}}$$ First, list down the values stated in the problemTotal length of the race `=` `77`km Length of a lap `=` `3 2/3`km Divide the two valuesStart by converting the mixed fraction to an improper fraction$$77\div\color{#00880A}{3}\frac{\color{#007DDC}{2}}{\color{#9a00c7}{3}}$$ `=` $$\frac{77}{1}\div\frac{(\color{#9a00c7}{3}\times\color{#00880A}{3})+\color{#007DDC}{2}}{\color{#9a00c7}{3}}$$ `=` `77/1-:(9+2)/3` `=` `77/1-:11/3` Get the reciprocal (flip) of the divisor`11/3` becomes `3/11` Proceed with multiplying this to the dividend.`77/1-:``11/3` `=` `77/1xx``3/11` `=` $$\frac{77\div\color{#CC0000}{11}}{1}\times\frac{3}{11\div\color{#CC0000}{11}}$$ Reduce the fractions `=` `7/1xx3/1` `=` `21/1` `=` `21` laps `21` laps -
Question 3 of 6
3. Question
How many `20`-minute periods are there in `5` hours?- (15) periods
Hint
Help VideoCorrect
Fantastic!
Incorrect
To divide fractions, flip the divisor and then multiply as normalMethod OneUse an illustration to represent the problem
`1` circle represents `1` hour or `60` minutes, so `5` circles are drawn.Since there are three `20` minutes in `60` minutes, each circle is divided into `3` parts.Count the total number of parts`5` circles `xx3` parts `=` `15` There are `15` `20`-minute periods in `5` hours`15` periodsMethod TwoFirst, list down the values stated in the problemTotal no. of hours `=` `5` hours Length of a period
(in hours)`=` `20` minutes `-:60` minutes`=20/60=1/3` Divide the two valuesStart by getting the reciprocal (flip) of the divisor`1/3` becomes `3/1` Proceed with multiplying this to the dividend.`5-:``1/3` `=` `5/1xx``3/1` `=` `15/1` `=` `15` There are `15` `20`-minute periods in `5` hours`15` periods -
Question 4 of 6
4. Question
Bill swims for exactly the same amount of time each day. If he swims for a total of `7 1/2` hours in `5` days, for how long did he swim each day?Hint
Help VideoCorrect
Excellent!
Incorrect
Transforming a Fraction from Mixed to Improper
`=` $$\frac{(\color{#9a00c7}{c}\times\color{#00880A}{A})+\color{#007DDC}{b}}{\color{#9a00c7}{c}}$$ Transforming an Improper to Mixed Fraction
$$\frac{\color{#007DDC}{b}}{\color{#9a00c7}{c}}=\color{#00880A}{Q}\frac{\color{#e65021}{R}}{\color{#9a00c7}{c}}$$`(``b``-:``c``)=``Q` and `R` is the remainderFirst, list down the values stated in the problemTotal no. of
swimming hours`=` `7 1/2` hours No. of days `=` `5` days Divide the two valuesStart by converting the mixed fraction to an improper fraction$$\color{#00880A}{7}\frac{\color{#007DDC}{1}}{\color{#9a00c7}{2}}\div5$$ `=` $$\frac{(\color{#9a00c7}{2}\times\color{#00880A}{7})+\color{#007DDC}{1}}{\color{#9a00c7}{2}}\div\frac{5}{1}$$ `=` `(14+1)/2-:5/1` `=` `15/2-:5/1` Get the reciprocal (flip) of the divisor`5/1` becomes `1/5` Proceed with multiplying this to the dividend.`15/2-:``5/1` `=` `15/2xx``1/5` `=` $$\frac{15\div\color{#CC0000}{5}}{2}\times\frac{1}{5\div\color{#CC0000}{5}}$$ Reduce the fractions `=` `3/2xx1/1` `=` `3/2` Convert the fraction from improper to mixedStart by dividing the numerator by the denominatorArrange the numbers for long division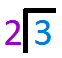
`2` goes into `3` once. So write `1` above the line.
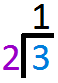Multiply `1` to `2` and write the answer below `3`
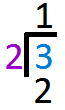Subtract `2` from `3` and write the answer one line below
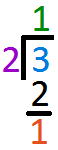Since `2` cannot go into `1` anymore, `1` is left as the Remainder and `1` is the QuotientSubstitute values into the given formula$$\frac{\color{#007DDC}{b}}{\color{#9a00c7}{c}}$$ `=` $$\color{#00880A}{Q}\frac{\color{#e65021}{R}}{\color{#9a00c7}{c}}$$ $$\frac{\color{#007DDC}{3}}{\color{#9a00c7}{2}}$$ `=` $$\color{#00880A}{1}\frac{\color{#e65021}{1}}{\color{#9a00c7}{2}}$$ Bill swam for `1 1/2` hours per day.`1 1/2` hours -
Question 5 of 6
5. Question
How many `1 1/3`m sections of cable can be cut from a `72`m roll?- (54) sections
Hint
Help VideoCorrect
Nice Job!
Incorrect
Transforming a Fraction from Mixed to Improper
`=` $$\frac{(\color{#9a00c7}{c}\times\color{#00880A}{A})+\color{#007DDC}{b}}{\color{#9a00c7}{c}}$$ First, list down the values stated in the problemTotal length of the rope `=` `72`m Sections it will be cut into `=` $$\color{#00880A}{1}\frac{\color{#007DDC}{1}}{\color{#9a00c7}{3}}=\frac{(\color{#9a00c7}{3}\times\color{#00880A}{1})+\color{#007DDC}{1}}{\color{#9a00c7}{3}}=\frac{3+1}{3}=\frac{4}{3}$$ Divide the two values`72-:4/3` `=` `72/1xx3/4` Reciprocate the divisor and change the operation to multiplication `=` $$\frac{72\div\color{#CC0000}{4}}{1}\times\frac{3}{4\div\color{#CC0000}{4}}$$ Reduce the fractions `=` `18/1xx3/1` Cancel diagonally `=` `54/1` `=` `54` A `72`m roll of cable can have `54` sections of `1 1/3`m.`54` -
Question 6 of 6
6. Question
A `$1600` TV has a `1/5` off discount. Find the following:-
`(i)` Discount: `$` (320)`(ii)` Sale Price: `$` (1280)
Hint
Help VideoCorrect
Well Done!
Incorrect
To multiply fractions, simply multiply the numerators and denominators separately.`(i)` Find the discount price of the TV.First, list down the values stated in the problemOriginal Price `=` `$1600` Discount Rate `=` `1/5` Multiply the two values`1600xx1/5` `=` `1600/1xx1/5` `=` `1600/5` `=` `1600-:5` `=` `$320` The discount price is `$320``(ii)` Find the sale price of the TV.First, list down the known values in the problemOriginal Price `=` `$1600` Discount Price `=` `$320` Subtract the two valuesOriginal price `-` Discount price `=` Sale price `$1600` `-` `$320` `=` `$1280` The sale price is `$1280``(i) $320``(ii) $1280` -
Quizzes
- Shaded Fractions 1
- Shaded Fractions 2
- Equivalent Fractions 1
- Equivalent Fractions 2
- Equivalent Fractions 3
- Equivalent Fractions 4
- Simplify Fractions 1
- Simplify Fractions 2
- Simplify Fractions 3
- Find the Lowest Common Denominator
- Comparing Fractions 1
- Comparing Fractions 2
- Comparing Fractions 3
- Mixed and Improper Fractions 1
- Mixed and Improper Fractions 2
- Mixed and Improper Fractions 3
- Add and Subtract Fractions 1
- Add and Subtract Fractions 2
- Add and Subtract Fractions 3
- Add and Subtract Fractions 4
- Multiply and Divide Fractions 1
- Multiply and Divide Fractions 2
- Multiply and Divide Fractions 3
- Add and Subtract Mixed Numbers 1
- Add and Subtract Mixed Numbers 2
- Add and Subtract Mixed Numbers 3
- Multiply and Divide Mixed Numbers 1
- Multiply and Divide Mixed Numbers 2
- Multiply and Divide Mixed Numbers 3
- Multiply and Divide Mixed Numbers 4
- Fraction Word Problems: Addition and Subtraction 1
- Fraction Word Problems: Addition and Subtraction 2
- Fraction Word Problems: Addition and Subtraction 3
- Fraction Word Problems: Addition and Subtraction 4
- Fraction Word Problems: Multiplication and Division
- Find the Fraction of a Quantity
- Find the Quantity of a Quantity 1
- Find the Quantity of a Quantity 2
- Find the Fraction of a Quantity: Word Problems 1
- Find the Fraction of a Quantity: Word Problems 2
- Find the Fraction of a Quantity: Word Problems 3
- Find the Fraction of a Quantity: Word Problems 4
- Find the Quantity of a Quantity: Word Problems
- Order of Operations Involving Fractions 1
- Order of Operations Involving Fractions 2