Years
>
Year 11>
Sequences and Series>
Arithmetic Sequence Problems>
Arithmetic Sequence ProblemsArithmetic Sequence Problems
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 3 questions completed
Questions:
- 1
- 2
- 3
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- Answered
- Review
-
Question 1 of 3
1. Question
Find the sequence given that:`U_5+U_13=126``U_9+U_17=182`Hint
Help VideoCorrect
Great Work!
Incorrect
General Rule of an Arithmetic Sequence
$$U_{\color{#9a00c7}{n}}=\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$First, transform the `5th` and `13th` terms into general rule form5th Term$$U_{\color{#9a00c7}{n}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$U_{\color{#9a00c7}{5}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{5}-1)\color{#00880A}{d}]$$ Substitute known values `U_5` `=` `a+4d` Distribute 13th Term$$U_{\color{#9a00c7}{n}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$U_{\color{#9a00c7}{13}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{13}-1)\color{#00880A}{d}]$$ Substitute known values `U_13` `=` `a+12d` Distribute Next, add the first pair general forms`U_5+U_13` `=` `(a+4d)+(a+12d)` `126``divide2` `=` `2a+16d``divide2` Divide both sides by `2` `63` `=` `a+8d` Next, transform the `9th` and `17th` terms into general rule form9th Term$$U_{\color{#9a00c7}{n}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$U_{\color{#9a00c7}{9}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{9}-1)\color{#00880A}{d}]$$ Substitute known values `U_9` `=` `a+8d` Distribute 17th Term$$U_{\color{#9a00c7}{n}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$U_{\color{#9a00c7}{17}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{17}-1)\color{#00880A}{d}]$$ Substitute known values `U_17` `=` `a+16d` Distribute Next, add the second pair general forms`U_9+U_17` `=` `(a+4d)+(a+12d)` `182``divide2` `=` `2a+24d``divide2` Divide both sides by `2` `91` `=` `a+12d` Next, solve for the value of `d` by subtracting the first combined general rule form from the second combined general rule form`91``-``63` `=` `(``a+12d``)-(``a+8d``)` `28``divide4` `=` `4d``divide4` Divide both sides by `4` `7` `=` `d` `d` `=` `7` Next, substitute `d` to one of the combined general rule forms to solve for `a``63` `=` `a+8``d` `63` `=` `a+8(``7``)` Substitute `d=7` `63` `-56` `=` `a+56` `-56` Subtract `56` from both sides `7` `=` `a` `a` `=` `7` Finally, start with `a=7` and keep adding `d=7` to its value to get the sequence`U_1` `=` `7` `U_2` `=` `7+``7` `=` `14` `U_3` `=` `14+``7` `=` `21` `U_4` `=` `21+``7` `=` `28` `7+14+21+28…` `7+14+21+28…` -
Question 2 of 3
2. Question
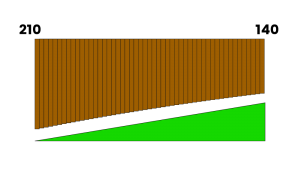
The short end of a fence has a height of `140` cm. After about `71` pieces of timber, the height of the fence is `210` cm. Find:`(i)` The difference in height between each fence`(ii)` The total height of the fences in metres
-
`(i)` `d=` (1)`\text(cm)``(ii)` `S_n=` (124.25)`\text(m)`
Hint
Help VideoCorrect
Nice Job!
Incorrect
Sum of an Arithmetic Sequence
$$S_{\color{#9a00c7}{n}}=\frac{\color{#9a00c7}{n}}{2}[2\color{#e65021}{a}+(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$General Rule of an Arithmetic Sequence
$$U_{\color{#9a00c7}{n}}=\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$`(i)` Finding the difference in height between each fence (`d`)Substitute the known values to the general rule`\text(Number of terms)``[n]` `=` `71` `\text(First term)``[a]` `=` `140` $$U_{\color{#9a00c7}{n}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$U_{\color{#9a00c7}{71}}$$ `=` $$\color{#e65021}{140}+[(\color{#9a00c7}{71}-1)\color{#00880A}{d}]$$ Substitute known values `210` `-140` `=` `140+70d` `-140` Subtract `140` from both sides `70``divide70` `=` `70d``divide70` Divide both sides by `70` `1` `=` `d` `d` `=` `1 \text(cm)` `(ii)` Finding the total height of the fences in metres (`S_n`)Substitute the known values to the sum formula`\text(Number of terms) [n]` `=` `71` `\text(First Term) [a]` `=` `140` `\text(Common Difference) [d]` `=` `1` $$S_{\color{#9a00c7}{n}}$$ `=` $$\frac{\color{#9a00c7}{n}}{2}[2\color{#e65021}{a}+(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$S_{\color{#9a00c7}{71}}$$ `=` $$\frac{\color{#9a00c7}{71}}{2}[2\cdot\color{#e65021}{140}+(\color{#9a00c7}{71}-1)\color{#00880A}{1}]$$ Substitute known values `=` $$35\frac{1}{2}(280+70)$$ Evaluate `=` $$35\frac{1}{2}(350)$$ `=` `12 425 \text(cm)` Finally, convert the centimetres into metres`1 \text(metre)` `=` `100 \text(centimetres)` `12425xx1/(100)` `=` `124.25 \text(cm)` `(i) d=1 \text(cm)``(ii) S_n=124.25 \text(m)` -
-
Question 3 of 3
3. Question
A stack of cans has `1` can at the top and then each row has `2` more cans than the previous row. Find:`(i)` The number of cans in the `21st` row`(ii)` The row with `61` cans`(iii)` The number of rows if there are a total of `1296` cans
-
`(i)` `U_21=` (41)`(ii)` `n=` (31)`(iii)` `n=` (36)
Hint
Help VideoCorrect
Well Done!
Incorrect
Sum of an Arithmetic Sequence
$$S_{\color{#9a00c7}{n}}=\frac{\color{#9a00c7}{n}}{2}[2\color{#e65021}{a}+(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$General Rule of an Arithmetic Sequence
$$U_{\color{#9a00c7}{n}}=\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$`(i)` Finding the number of cans in the `21st` row (`U_21`)Substitute the known values to the general rule`\text(Number of terms)``[n]` `=` `21` `\text(First term)``[a]` `=` `1` `\text(Common Difference)``[d]` `=` `2` $$U_{\color{#9a00c7}{n}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$U_{\color{#9a00c7}{21}}$$ `=` $$\color{#e65021}{1}+[(\color{#9a00c7}{21}-1)\color{#00880A}{2}]$$ Substitute known values `=` `1+(20)2` Evaluate `=` `1+40` `=` `41` `(ii)` Finding the row with `61` cans (`n`)Substitute the known values to the general rule and solve for `n``\text(Nth term)``[U_n]` `=` `61` `\text(First term)``[a]` `=` `1` `\text(Common Difference)``[d]` `=` `2` $$U_{\color{#9a00c7}{n}}$$ `=` $$\color{#e65021}{a}+[(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$61$$ `=` $$\color{#e65021}{1}+[(\color{#9a00c7}{n}-1)\color{#00880A}{2}]$$ Substitute known values `61` `=` `1+(2n-2)` Distribute `61` `+1` `=` `-1+2n` `+1` Add `1` to both sides `62``divide2` `=` `2n``divide2` Divide both sides by `2` `31` `=` `n` `n` `=` `31` `(iii)` Finding the number of rows if `S_n=1296` (`n`)Substitute the known values to the sum formula and solve for `n``\text(Sum of terms)``[S_n]` `=` `1296` `\text(First Term) [a]` `=` `1` `\text(Common Difference) [d]` `=` `2` $$S_{\color{#9a00c7}{n}}$$ `=` $$\frac{\color{#9a00c7}{n}}{2}[2\color{#e65021}{a}+(\color{#9a00c7}{n}-1)\color{#00880A}{d}]$$ $$1296$$ `=` $$\frac{\color{#9a00c7}{n}}{2}[2\cdot\color{#e65021}{1}+(\color{#9a00c7}{n}-1)\color{#00880A}{2}]$$ Substitute known values `1296` `=` $$\frac{n}{2}(2+2n-2)$$ Evaluate `1296``times2` `=` `[n/2(2n)]``times2` Multiply both sides by `2` `2592` `=` `n(2n)` `2/2=1` `2592``divide2` `=` `2n^2``divide2` Divide both sides by `2` `sqrt1296` `=` `sqrt(n^2)` Find the square root of both sides `36` `=` `n` `n` `=` `36` `(i) U_21=41``(ii) n=31``(iii) n=36` -