Solving for Bearings
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 7 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- Answered
- Review
-
Question 1 of 7
1. Question
Find the bearing of C from A- AC= (124)°T
Hint
Help VideoCorrect
Well Done!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Sin Ratio
sin=oppositehypotenuseCos Ratio
cos=adjacenthypotenuseTan Ratio
tan=oppositeadjacentA true bearing is an angle measured clockwise from the True North around to the required direction.Notice that when starting from North and heading to East, the two lines form a complementary angle.Since complementary angles add up to 90°, we find the value of θ and add it to 90° to get the true bearing of C from A.To solve for θ, we can use the known values that are opposite and adjacent to it.Since we have the opposite and adjacent values, we can solve for tanθtanθ = oppositeadjacent tanθ = 46 tanθ = 0.6666… Remember that we are looking for θ, not tanθUse your calculator to find the value of θ. The common key combinations on your calculator would be:Shift +tan+0.6666… or tan-1+0.6666… This will give you the value of 33.69° or 33°41’, depending on the calculator.Next, round the value to the nearest degree. For rounding values:Degrees with decimals:Decimal value below 50: Round downDecimal value 50 or above: Round upDegrees with minutes:Minute value below 30: Round downMinute value 30 or above: Round upThis will give us θ=34°.Finally, add 34° to 90° to find the true bearing of C from A.AC = 90+34 = 124°T AC=124°T -
Question 2 of 7
2. Question
Bianca leaves her home and cycles due north for 12km, then 7km due west to the gym. What is the bearing of her home from the gym?- HG= (150)°T
Hint
Help VideoCorrect
Nice Job!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Sin Ratio
sin=oppositehypotenuseCos Ratio
cos=adjacenthypotenuseTan Ratio
tan=oppositeadjacentA true bearing is an angle measured clockwise from the True North around to the required direction.Notice that when starting from North and heading to East, the two lines form a complementary angle.Since complementary angles add up to 90°, we find the value of θ and add it to 90° to get the true bearing of H from G.To solve for θ, we can use the known values that are opposite and adjacent to it.Since we have the opposite and adjacent values, we can solve for tanθtanθ = oppositeadjacent tanθ = 127 Remember that we are looking for θ, not tanθUse your calculator to find the value of θ. The common key combinations on your calculator would be:Shift +tan+(127) or tan-1+(127) This will give you the value of 59.74° or 59°45’, depending on the calculator.Next, round the value to the nearest degree. For rounding values:Degrees with decimals:Decimal value below 50: Round downDecimal value 50 or above: Round upDegrees with minutes:Minute value below 30: Round downMinute value 30 or above: Round upThis will give us θ=60°.Finally, add 60° to 90° to find the true bearing of H from G.HG = 90+60 = 150°T HG=150°T -
Question 3 of 7
3. Question
Town A is 128 km east and 165 km south of town B. Find the true bearing of town A from town B.Round your answer to the nearest minute- BA= (142)° (12)′T
Hint
Help VideoCorrect
Excellent!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Sin Ratio
sin=oppositehypotenuseCos Ratio
cos=adjacenthypotenuseTan Ratio
tan=oppositeadjacentA true bearing is an angle measured clockwise from the True North around to the required direction.Notice that when starting from North and heading to East, the two lines form a complementary angle.Since complementary angles add up to 90°, we find the value of θ and add it to 90° to get the true bearing of C from A.To solve for θ, we can use the known values that are opposite and adjacent to it.Since we have the opposite and adjacent values, we can solve for tanθtanθ = oppositeadjacent tanθ = 165128 tanθ = 1.289 Remember that we are looking for θ, not tanθUse your calculator to find the value of θ. The common key combinations on your calculator would be:Shift +tan+1.289 or tan-1+1.289 This will give you the value of 52.1972° or 52°11’50″, depending on the calculator.Since we are looking for the value with the nearest degree, convert 52.1972° to Degrees-Minute-Second form by pressing the DMS button on a calculator.Next, round the value to the nearest degree. For rounding values:Degrees with minutes:Minute/Second value below 30: Round downMinute/Second value 30 or above: Round upThis will give us θ=52°12’.Finally, add 52°12’ to 90° to find the true bearing of A from B.BA = 90°+52°12’ = 142°12’T BA=142°12’°T -
Question 4 of 7
4. Question
Town X is 87 km west and 29 km north of town Y. Find the compass bearing of town X from town Y.- XY= (N) (72)° (W)
Hint
Help VideoCorrect
Fantastic!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Sin Ratio
sin=oppositehypotenuseCos Ratio
cos=adjacenthypotenuseTan Ratio
tan=oppositeadjacentTo get a compass bearing, take the compass quadrant where the direction belongs to, then get the angle from the vertical line around to the required direction.
Compass Bearing Quadrants
Northwest Quadrant=N __ WNortheast Quadrant=N __ ESouthwest Quadrant=S __ WSoutheast Quadrant=S __ EThe blank is filled with the distance of the bearing from the North-South (vertical) lineSince we are looking for the compass bearing, we need to find the value of the angle spanning from the North line. Mark it as θ.To solve for θ, we can use the known values that are opposite and adjacent to it.Since we have the opposite and adjacent values, we can solve for tanθtanθ = oppositeadjacent tanθ = 8729 tanθ = 3 Remember that we are looking for θ, not tanθUse your calculator to find the value of θ. The common key combinations on your calculator would be:Shift +tan+3 or tan-1+3 This will give you the value of 71.56505° or 71°34’, depending on the calculator.Next, round the value to the nearest degree. For rounding values:Degrees with minutes:Minute/Second value below 30: Round downMinute/Second value 30 or above: Round upThis will give us θ=72°.Finally, notice that town X is located on the Northwest Quadrant.Using the chart, we can write the compass bearing of X from Y by using N __ W. Then get the angle that spans from the vertical line (N) to X, which is 72°Therefore, the compass bearing of X from Y is N 72°WXY=N 72°W -
Question 5 of 7
5. Question
Town A is 420 km west and 760 km north of town B. Find the true bearing of town A from town B.Round your answer to the nearest degree- AB= (331)°T
Hint
Help VideoCorrect
Keep Going!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Sin Ratio
sin=oppositehypotenuseCos Ratio
cos=adjacenthypotenuseTan Ratio
tan=oppositeadjacentA true bearing is an angle measured clockwise from the True North around to the required direction.Notice that when starting from North and moving clockwise to line A, it passes through 3 quadrants which are 90° each.Now, to solve for θ, we can use the known values that are opposite and adjacent to it.Since we have the opposite and adjacent values, we can solve for tanθtanθ = oppositeadjacent tanθ = 760420 tanθ = 1.80952 Remember that we are looking for θ, not tanθUse your calculator to find the value of θ. The common key combinations on your calculator would be:Shift +tan+1.80952 or tan-1+1.80952 This will give you the value of 61.07° or 61°04’, depending on the calculator.Next, round the value to the nearest degree. For rounding values:Degrees with decimals:Decimal value below 50: Round downDecimal value 50 or above: Round upDegrees with minutes:Minute value below 30: Round downMinute value 30 or above: Round upThis will give us θ=61°.Finally, add the angles that span from the North line clockwise to line A to find the true bearing of A from B.AB = 90+90+90+61 AB = 270+61 = 331°T AB=331°T -
Question 6 of 7
6. Question
Find the bearing of B from C- BC= (310)°T
Hint
Help VideoCorrect
Exceptional!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
A true bearing is an angle measured clockwise from the True North around to the required direction.Notice that when starting from the North line on the right and heading clockwise to line AC, we go around three quadrants, which are 90° each.This means we can simply add those three 90° angles to 40°Don’t forget to add T to the bearing to indicate that it’s true North.BC = 90+90+90+40 = 270+40 = 310°T BC=310°T -
Question 7 of 7
7. Question
Find the true bearing of M from KRound your answer to the nearest minute- M from K= (198)° (8)′T
Hint
Help VideoCorrect
Correct!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
Sin Ratio
sin=oppositehypotenuseCos Ratio
cos=adjacenthypotenuseTan Ratio
tan=oppositeadjacentA true bearing is an angle measured clockwise from the True North around to the required direction.Co-Interior Angles are when two angles have a sum of 180°.First, find the value of angle MKL. Label it as θ.Determine that angle KLM is a right angle.180°-35°-55° = 90° To solve for θ, we can use the known values that are opposite and adjacent to it.Since we have the opposite and adjacent values, we can solve for tanθtanθ = oppositeadjacent tanθ = 86 Remember that we are looking for θ, not tanθUse your calculator to find the value of θ. The common key combinations on your calculator would be:Shift +tan+(86) or tan-1+(86) This will give you the value of 53.13° or 53°7’48″, depending on the calculator.Since we are looking for the value with the nearest degree, convert 53.13° to Degrees-Minute-Second form by pressing the DMS button on a calculator.Next, round the value to the nearest degree. For rounding values:Degrees with minutes:Minute/Second value below 30: Round downMinute/Second value 30 or above: Round upThis will give us θ=53°8’.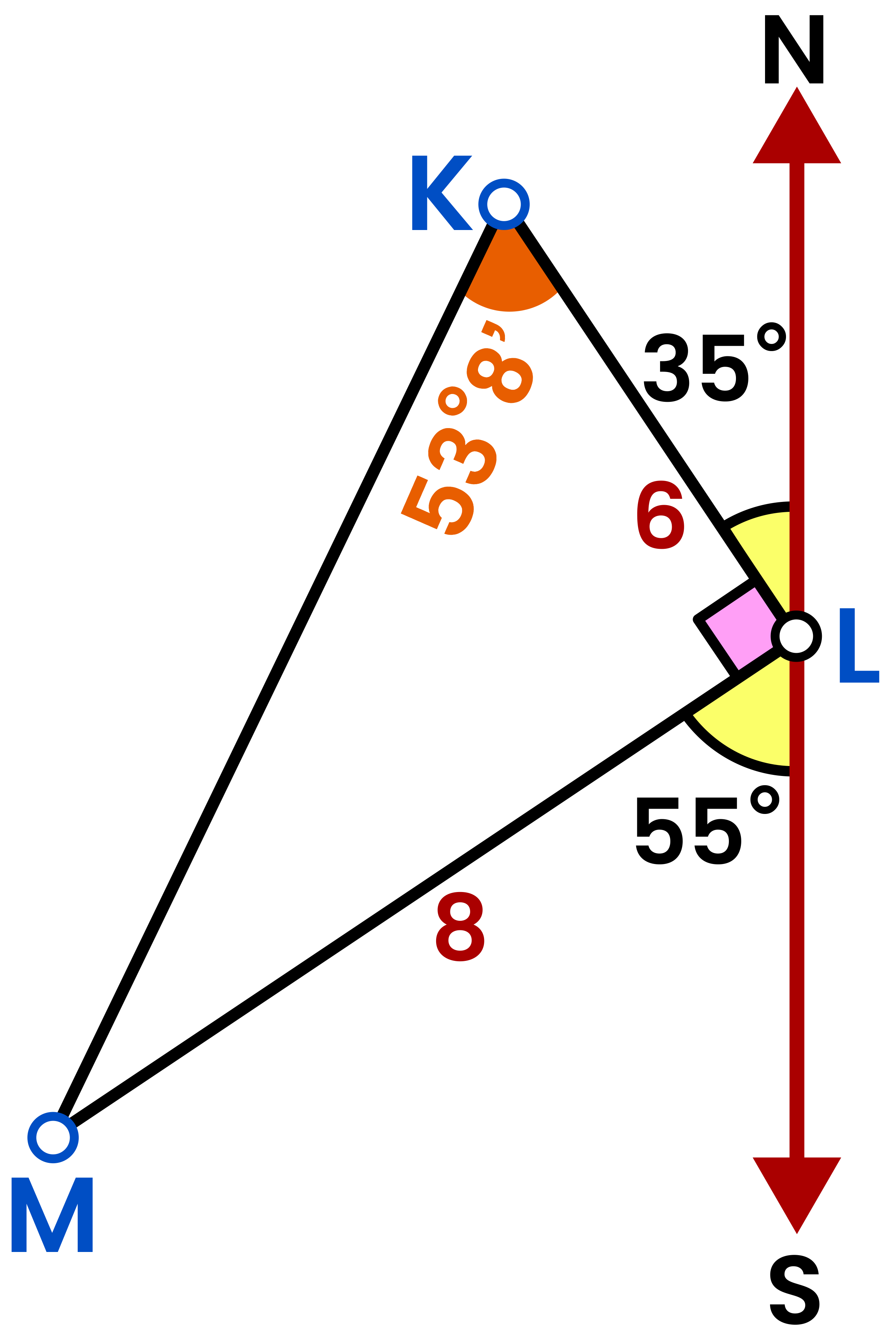 Next, draw a cross hair for point K since this is where we are getting the bearing from.
Next, draw a cross hair for point K since this is where we are getting the bearing from.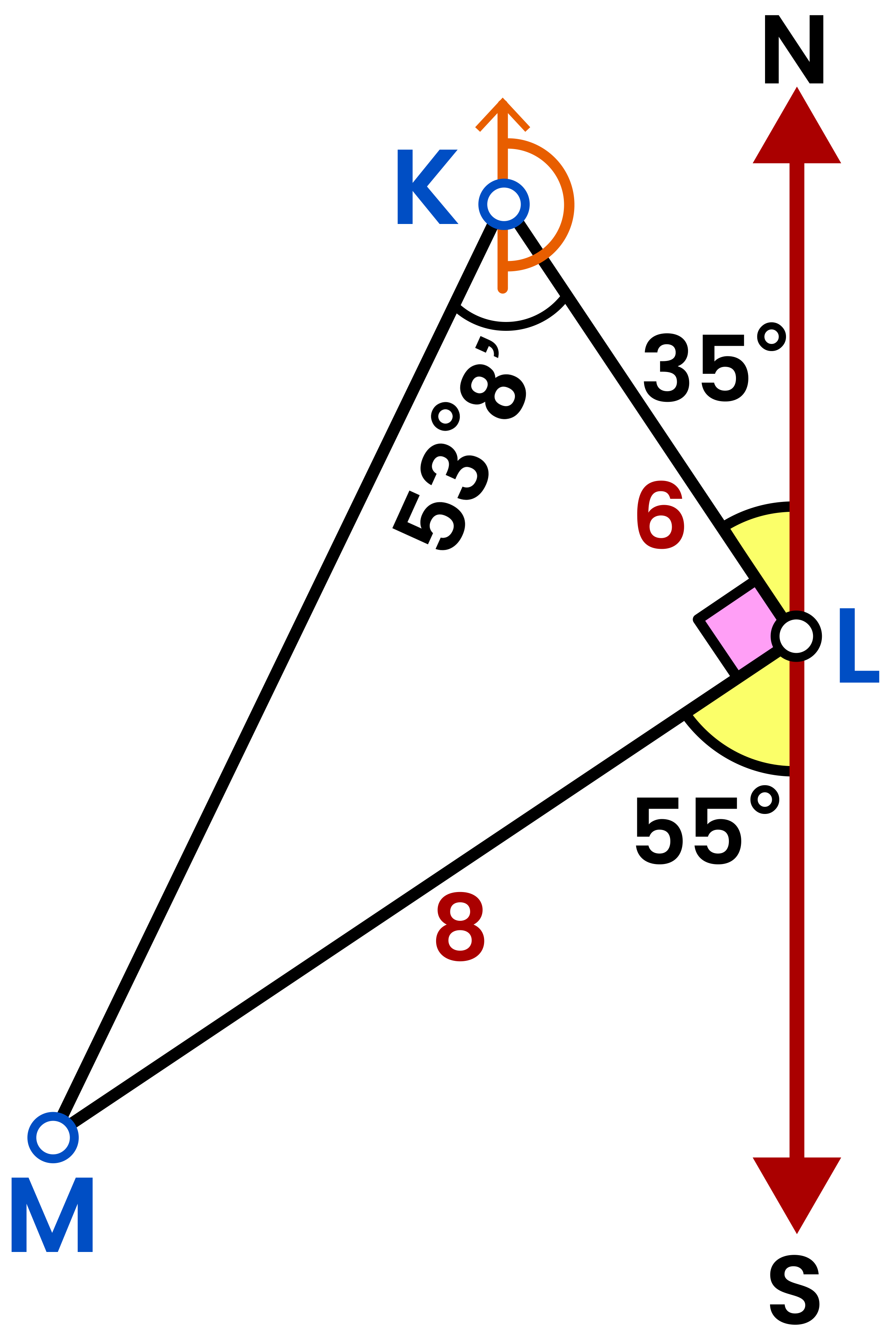 Notice that the remaining missing angle and angle NLK are co-interior angles.Since co-interior angles sum up to 180°, we can simply subtract 35° from 180° to get the value of the missing angle.
Notice that the remaining missing angle and angle NLK are co-interior angles.Since co-interior angles sum up to 180°, we can simply subtract 35° from 180° to get the value of the missing angle.180°-35° = 145° 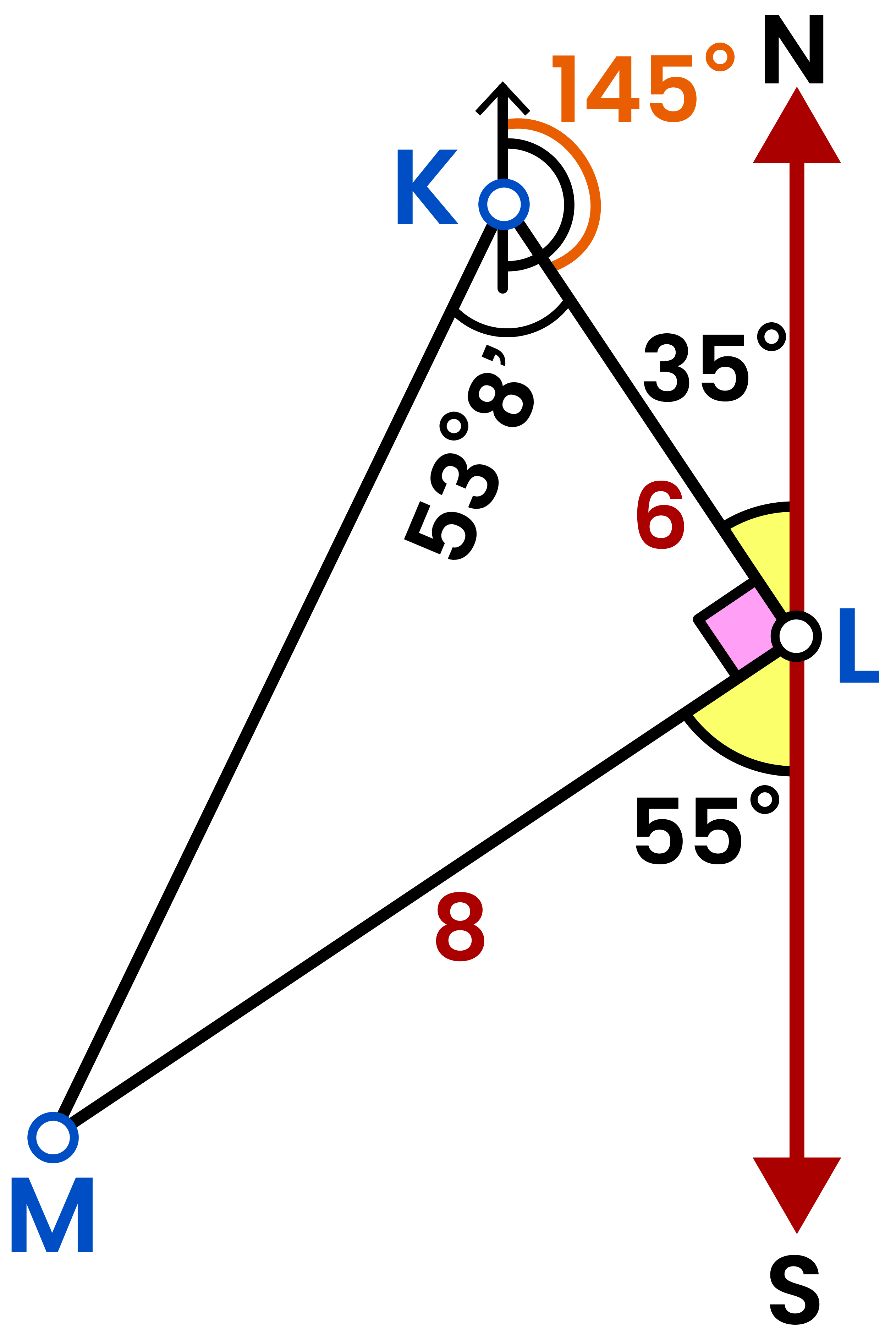 Finally, add 145° to the value of θ, which is 53°8’, to find the true bearing of M from K.
Finally, add 145° to the value of θ, which is 53°8’, to find the true bearing of M from K.MK = 53°8’+145° = 198°8’T M from K=198°8’T
Quizzes
- Compass Bearings and True Bearings 1
- Compass Bearings and True Bearings 2
- Solving for Bearings
- Bearings from Opposite Direction
- Using Bearings to Find Distance 1
- Using Bearings to Find Distance 2
- Using Bearings to Find Distance 3
- Using Bearings and Distances to Find Angles
- Working with Radial Surveys 1
- Working with Radial Surveys 2
- Working with Radial Surveys 3
- Working with Radial Surveys 4


































