>
Year 9>
Simultaneous Equations>
Solve Simultaneous Equations by Graphing>
Solve Simultaneous Equations by GraphingSolve Simultaneous Equations by Graphing
Try VividMath Premium to unlock full access
Quiz summary
0 of 7 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- Answered
- Review
-
Question 1 of 7
1. Question
Find the solution to the simultaneous equations by graphing.
x-3=0x−3=0
x+y=1x+y=1
- x=x= (3) y=y= (-2)
Correct
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
First graph the equations.Graph: x-3=0x−3=0Make xx the subject to get the equation of the line.x-3 +3x−3 +3 == 0 +30 +3 Add 33 to both sides x -3+3x −3+3 == 0 +30 +3 xx == 33 Graph: x+y=1x+y=1First, make yy the subject.x+yx+y == 11 yy == -x+1−x+1 Make yy the subject Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy 11 00 -1−1 -2−2 Plot the points and draw the graph.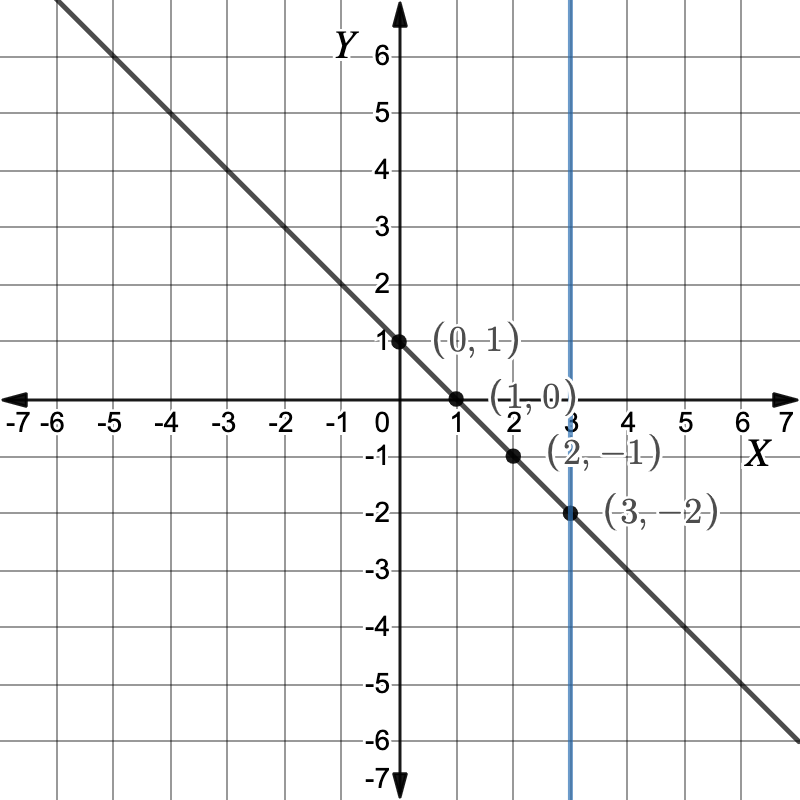 The graphs intersect at the point (3,-2)(3,−2)∴x=3, y=-2∴x=3, y=−2x=3,y=-2x=3,y=−2
The graphs intersect at the point (3,-2)(3,−2)∴x=3, y=-2∴x=3, y=−2x=3,y=-2x=3,y=−2 -
Question 2 of 7
2. Question
Find the solution to the simultaneous equations by graphing.
2x+y=52x+y=5
5x-3y=75x−3y=7
- x=x= (2) y=y= (1)
Correct
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
First graph the equations.Graph: 2x+y=52x+y=5Make yy the subject to get the equation of the line.2x+y -2x2x+y −2x == 5 -2x5 −2x Add -2x−2x to both sides y +2x-2xy +2x−2x == 5 -2x5 −2x yy == -2x+5−2x+5 Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy 55 33 11 -1−1 Plot the points and draw the graph.Graph: 5x-3y=75x−3y=7First, make yy the subject.5x-3y5x−3y == 77 Make yy the subject -3y−3y == -5x+7−5x+7 Add -5x−5x to both sides yy == 53x-7353x−73 Divide both sides by -3−3 Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy -73−73 -23−23 11 8383 Plot the points and draw the graph.The graphs intersect at the point (2,1)(2,1)∴x=2, y=1∴x=2, y=1x=2,y=1x=2,y=1 -
Question 3 of 7
3. Question
Find the solution to the simultaneous equations by graphing.
4x-3y=14x−3y=1
3x+2y=53x+2y=5
- x=x= (1) y=y= (1)
Correct
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
First graph the equations.Graph: 4x-3y=14x−3y=1Make yy the subject to get the equation of the line.4x-3y -4x4x−3y −4x == 1 -4x1 −4x Add -4x−4x to both sides -3y +4x-4x−3y +4x−4x == 1 -4x1 −4x -3y ÷(-3) −3y ÷(−3) == (-4x+1) ÷(-3)(−4x+1) ÷(−3) Divide both sides by -3−3 yy == 43x-1343x−13 Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy -13−13 11 7373 113113 Plot the points and draw the graph.Graph: 3x+2y=53x+2y=5First, make yy the subject.3x+2y3x+2y == 55 Make yy the subject 2y2y == -3x+5−3x+5 Add -3x−3x to both sides yy == -32x+52−32x+52 Divide both sides by 22 Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy 5252 11 -12−12 -2−2 Plot the points and draw the graph.The graphs intersect at the point (1,1)(1,1)∴x=1, y=1∴x=1, y=1x=1,y=1x=1,y=1 -
Question 4 of 7
4. Question
Find the solution to the simultaneous equations by graphing.
x+3y=15x+3y=15
y-x=1y−x=1
- x=x= (3) y=y= (4)
Correct
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
First graph the equations.Graph: x+3y=15x+3y=15Make yy the subject to get the equation of the line.x+3y -xx+3y −x == 15 -x15 −x Add -x−x to both sides 3y +x-x3y +x−x == 15 -x15 −x 3y ÷(3) 3y ÷(3) == (-x+15) ÷(3)(−x+15) ÷(3) Divide both sides by 33 yy == -13x+5−13x+5 Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy 55 143143 133133 44 Plot the points and draw the graph.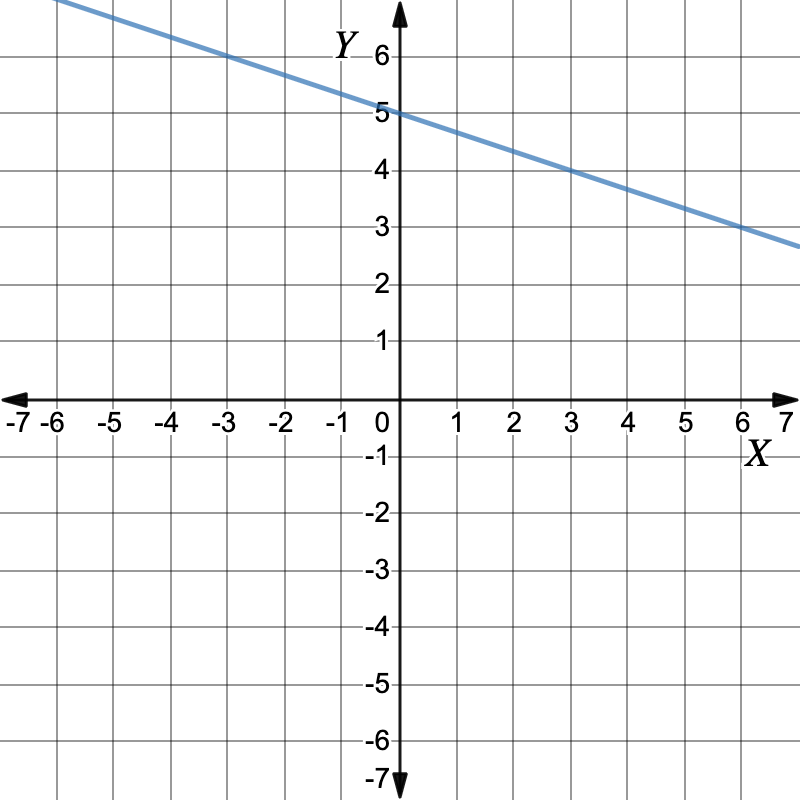 Graph: y-x=1y−x=1First, make yy the subject.
Graph: y-x=1y−x=1First, make yy the subject.y-xy−x == 11 yy == x+1x+1 Make yy the subject Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy 11 22 33 44 Plot the points and draw the graph.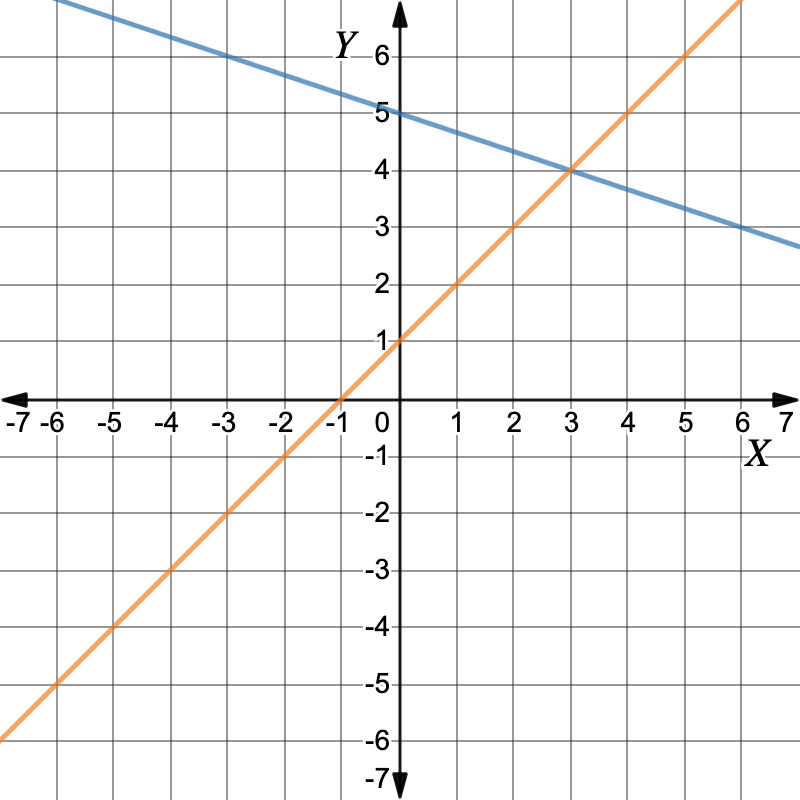 The graphs intersect at the point (3,4)(3,4)
The graphs intersect at the point (3,4)(3,4)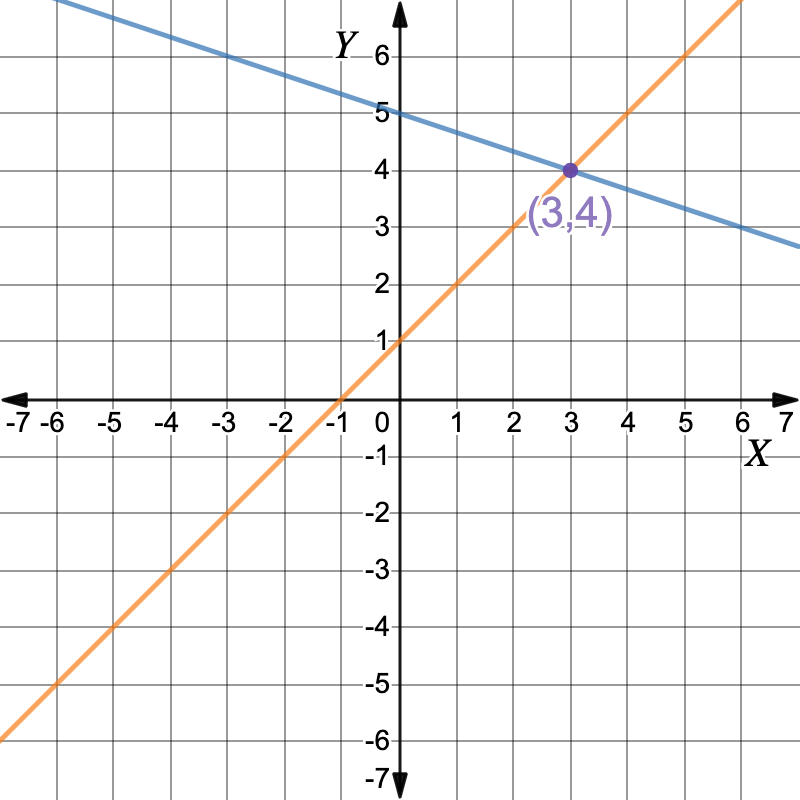 ∴x=3, y=4∴x=3, y=4x=3,y=4x=3,y=4
∴x=3, y=4∴x=3, y=4x=3,y=4x=3,y=4 -
Question 5 of 7
5. Question
Find the solution to the simultaneous equations by graphing.
2x+y=22x+y=2
x+y=2x+y=2
- x=x= (0) y=y= (2)
Correct
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
First graph the equations.Graph: 2x+y=22x+y=2Make yy the subject to get the equation of the line.2x+y -2x2x+y −2x == 2 -2x2 −2x Add -2x−2x to both sides y +2x-2xy +2x−2x == 2 -2x2 −2x yy == -2x+2−2x+2 Working out the y-values for each x-value in the table of values.xx 00 11 22 33 yy 22 00 -2−2 -4−4 Plot the points and draw the graph.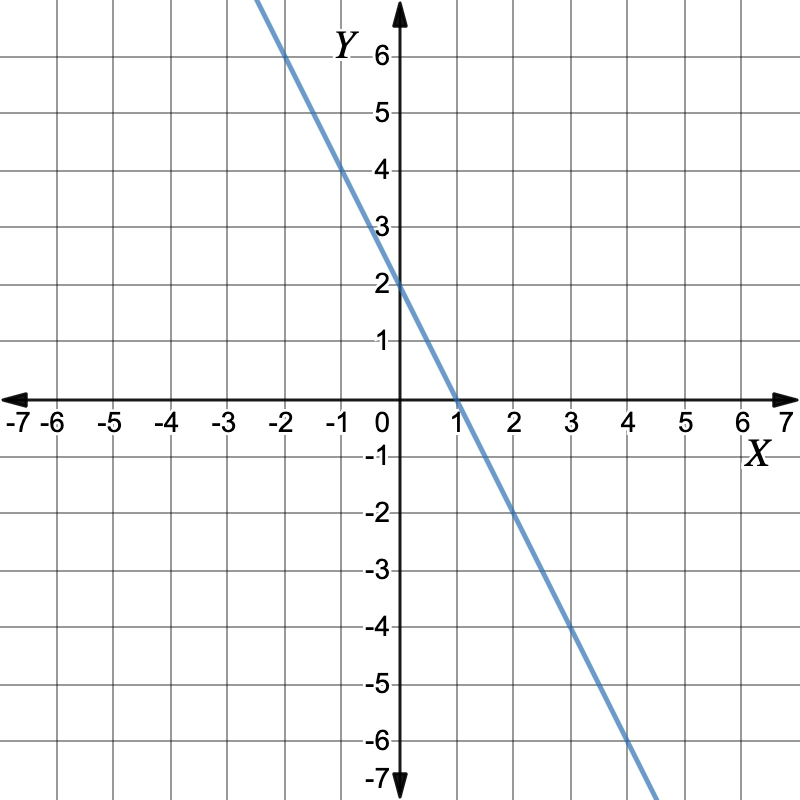 Graph: x+y=2x+y=2First, make yy the subject.
Graph: x+y=2x+y=2First, make yy the subject.x+yx+y == 22 yy == -x+2−x+2 Make y the subject Working out the y-values for each x-value in the table of values.x 0 1 2 3 y 2 1 0 -1 Plot the points and draw the graph.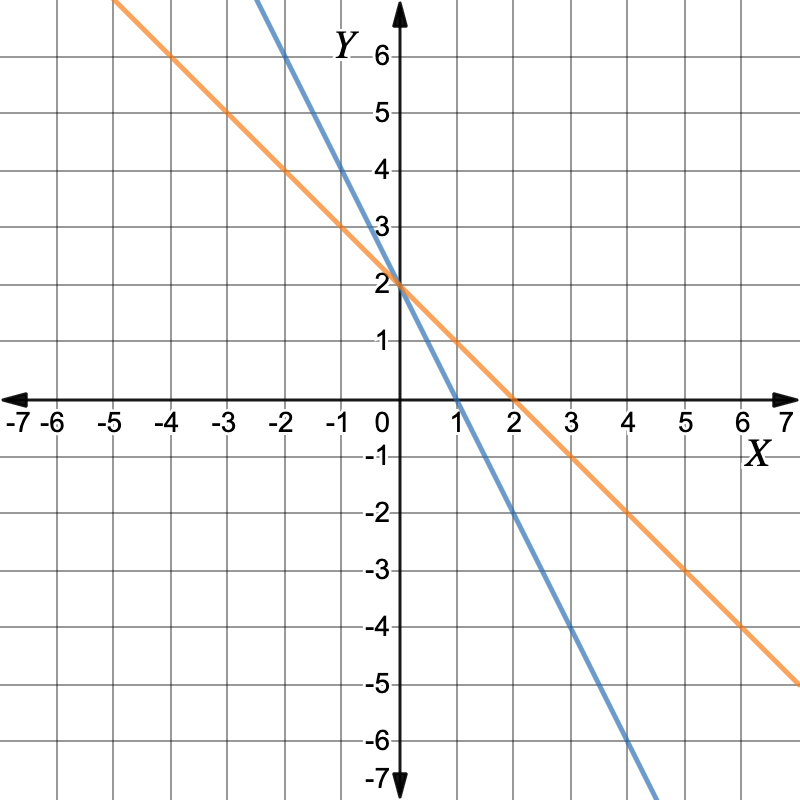 The graphs intersect at the point (0,2)
The graphs intersect at the point (0,2)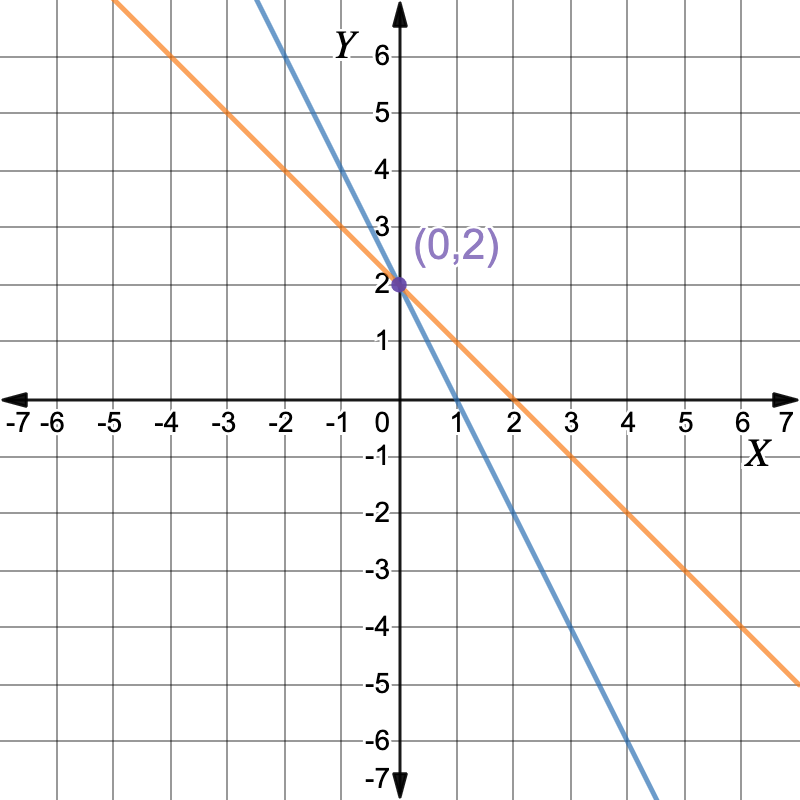 ∴x=0, y=2x=0,y=2
∴x=0, y=2x=0,y=2 -
Question 6 of 7
6. Question
Find the solution to the simultaneous equations by graphing.
y-x=4
x-y=1
- x= (no solution) y= (no solution)
Correct
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
First graph the equations.Graph: y-x=4Make y the subject to get the equation of the line.y-x +x = 4 +x Add x to both sides y -x+x = 4 +x y = x+4 Working out the y-values for each x-value in the table of values.x -2 0 2 4 y 2 4 6 8 Plot the points and draw the graph.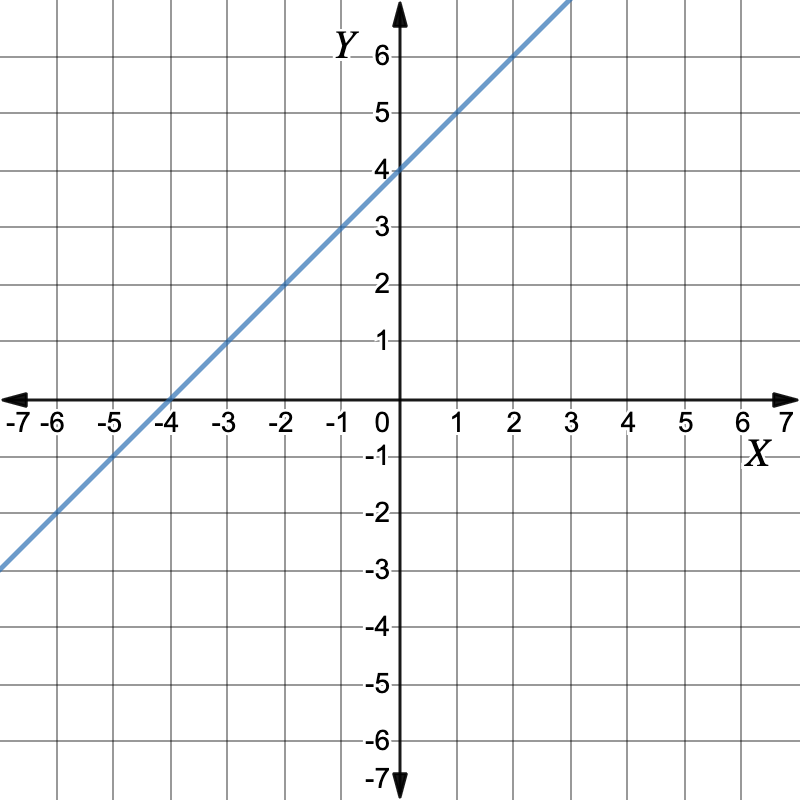 Graph: x+y=1First, make y the subject.
Graph: x+y=1First, make y the subject.x-y = 1 y = x-1 Make y the subject Working out the y-values for each x-value in the table of values.x -2 0 2 4 y -3 -1 1 3 Plot the points and draw the graph.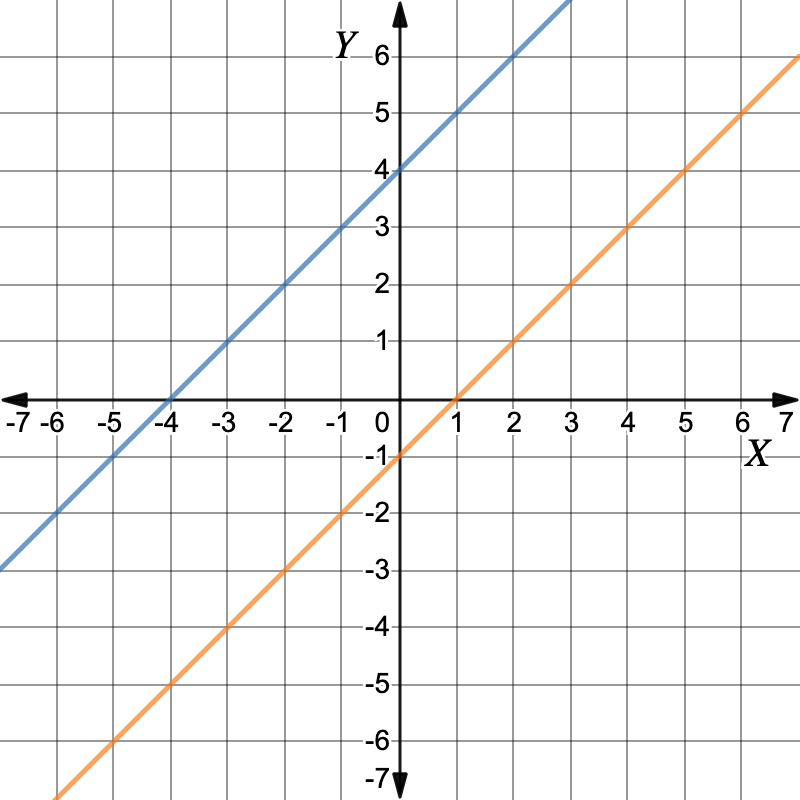 The graphs are parallel the lines do not intersect.There is no solution.There is no solution.
The graphs are parallel the lines do not intersect.There is no solution.There is no solution. -
Question 7 of 7
7. Question
Find the solution to the simultaneous equations by graphing.
-6x+2y=8
y=3x+4
- x= (infinite solutions) y= (infinite solutions)
Correct
Great Work!
Incorrect
Need TextPlayCurrent Time 0:00/Duration Time 0:00Remaining Time -0:00Stream TypeLIVELoaded: 0%Progress: 0%0:00Fullscreen00:00MutePlayback Rate1x- 2x
- 1.5x
- 1.25x
- 1x
- 0.75x
- 0.5x
Subtitles- subtitles off
Captions- captions off
- English
Chapters- Chapters
First graph the equations.Graph: -6x+2y=8Make y the subject to get the equation of the line.-6x+2y +6x = 8 +6x Add 6x to both sides 2y -6x+6x = 8 +6x 2y ÷(2) = (6x+8) ÷(2) Divide both sides by 2 y = 3x+4 Working out the y-values for each x-value in the table of values.x -2 -1 0 1 y -2 1 4 7 Plot the points and draw the graph.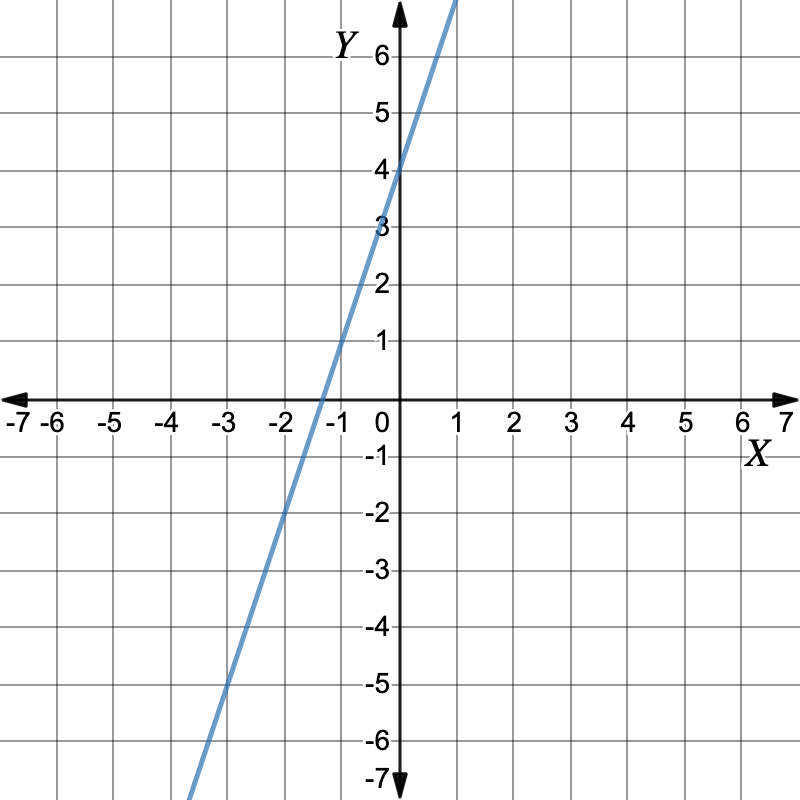 Graph: y=3x+4First, y is the subject.
Graph: y=3x+4First, y is the subject.y = 3x+4 y is the subject Working out the y-values for each x-value in the table of values.x -2 -1 0 1 y -2 1 4 7 Plot the points and draw the graph.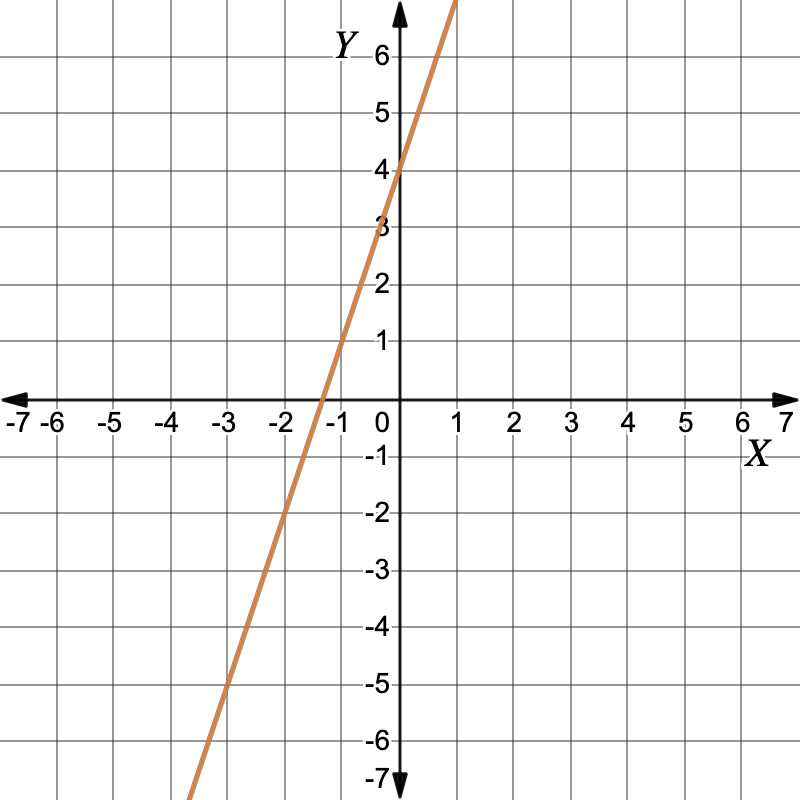 The graphs are both the same single line. This means there is an infinite number of solutions.Infinite solutionsInfinite solutions
The graphs are both the same single line. This means there is an infinite number of solutions.Infinite solutionsInfinite solutions
Quizzes
- Solve Simultaneous Equations by Graphing
- Substitution Method 1
- Substitution Method 2
- Substitution Method 3
- Substitution Method 4
- Elimination Method 1
- Elimination Method 2
- Elimination Method 3
- Elimination Method 4
- Nonlinear Simultaneous Equations
- Simultaneous Equations Word Problems 1
- Simultaneous Equations Word Problems 2
- 3 Variable Simultaneous Equations – Substitution Method
- 3 Variable Simultaneous Equations – Elimination Method











