Working with Radial Surveys 4
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 4 questions completed
Questions:
- 1
- 2
- 3
- 4
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- 4
- Answered
- Review
-
Question 1 of 4
1. Question
From the radial survey below, find `anglePOQ`: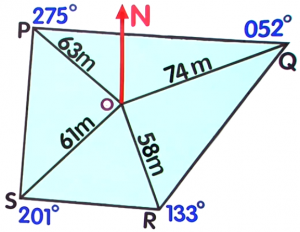
- `anglePOQ=` (137)`°`
Hint
Help VideoCorrect
Correct!
Incorrect
A radial survey is a tool used for land and seafloor mapping. Each corner of the area being measured is connected to a central point.Since `anglePOQ` is the sum of `anglePON` and `angleNOQ`, start with solving for `anglePON`.Knowing that a full revolution from `N` measures `360°`, subtract the bearing of `P` to get `anglePON``anglePON` `=` `360°-angleP` `=` `360°-275°` Substitute the values `=` `85°`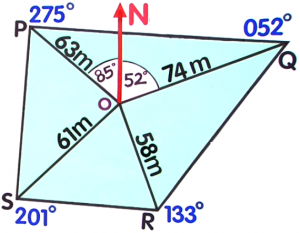 
Finally, proceed with adding `anglePON` and `angleNOQ`.`anglePOQ` `=` `anglePON+angleNOQ` `=` `85°+52°` Substitute the values `=` `137°`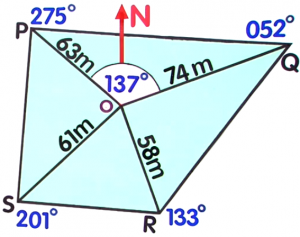 
`137°` -
Question 2 of 4
2. Question
From the radial survey below, find the area of `trianglePOQ` to the nearest square metre.- Area of `trianglePOQ=` (1590)`m^2`
Hint
Help VideoCorrect
Exceptional!
Incorrect
Area of a Non-Right Angled Triangle
`A_triangle=1/2``a``b``sin``C`where:
`a` is the side opposite angle `A`
`b` is the side opposite angle `B`
`c` is the side opposite angle `C`A radial survey is a tool used for land and seafloor mapping. Each corner of the area being measured is connected to a central point.First, identify the known values of the triangle `POQ`.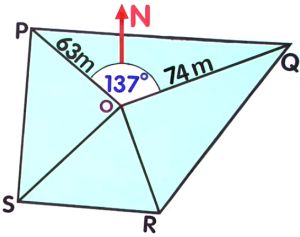
Now, substitute the known values to the formula and solve for the area.
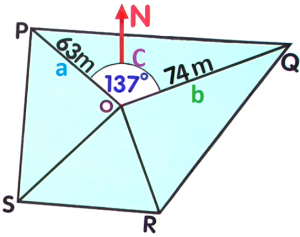`a=63m``b=74m``C=137°``A_triangle` `=` `1/2``a``b``sin``C` `=` `1/2(``63``)(``74``)sin``137°` Evaluate `sin` `137` on your calculator `=` `2331times0.68199836°` Simplify `=` `1589.74` `=` `1590m^2` Round off to the nearest metre `1590m^2` -
Question 3 of 4
3. Question
From the radial survey below, find `angleSOR`: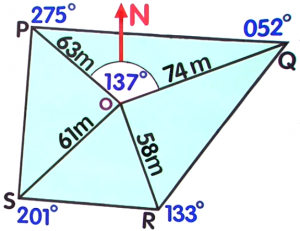
- `angleSOR=` (68)`°`
Hint
Help VideoCorrect
Keep Going!
Incorrect
A radial survey is a tool used for land and seafloor mapping. Each corner of the area being measured is connected to a central point.Notice that `angleSOR` is the difference between the bearings of `S` and `R`.Subtract the bearing of `R` from the bearing of `S`.`angleSOR` `=` `angleS-angleR` `=` `201°-133°` Substitute the values `=` `68°`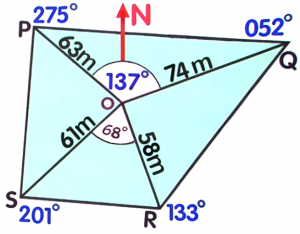 
`68°` -
Question 4 of 4
4. Question
From the radial survey below, find the length of `SR` to the nearest metre.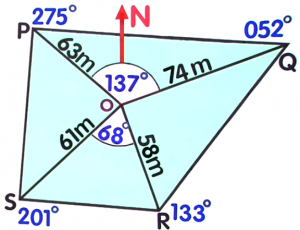
- `SR=` (67)`m`
Hint
Help VideoCorrect
Fantastic!
Incorrect
Cosine Law
$$\color{#007DDC}{a}^2=\color{#00880A}{b}^2+\color{#9a00c7}{c}^2-2\color{#00880A}{b}\color{#9a00c7}{c}\cos\color{#007DDC}{A}$$where:
`a` is the side opposite angle `A`
`b` is the side opposite angle `B`
`c` is the side opposite angle `C`Since `2` sides are given together with an angle between them, use the Cosine Law.First, label the triangle according to the Cosine Law.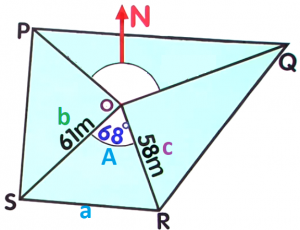
Substitute the three known values to the Cosine Law to find the length of side `SR` or `a`.From labelling the triangle, we know that the known values are those with labels `A, b` and `c`.`A=68°``b=61m``c=58m`$$\color{#007DDC}{a}^2$$ `=` $$\color{#00880A}{b}^2+\color{#9a00c7}{c}^2-2\color{#00880A}{b}\color{#9a00c7}{c}\cos\color{#007DDC}{A}$$ $$\color{#007DDC}{a}^2$$ `=` $$\color{#00880A}{61}^2+\color{#9a00c7}{58}^2-2(\color{#00880A}{61})(\color{#9a00c7}{58})\cos\color{#007DDC}{68°}$$ Substitute the values `a^2` `=` `3721+3364-7076cos68°` Evaluate `cos` `68` on your calculator `a^2` `=` `7085-7076(0.37460659)` `a^2` `=` `7085-2650.7162` `a^2` `=` `4434.283769` `sqrt(a^2)` `=` `sqrt4434.283769` Take the square root of both sides `a` `=` `66.59m` `a` or `BC` `=` `67m` Round off to the nearest metre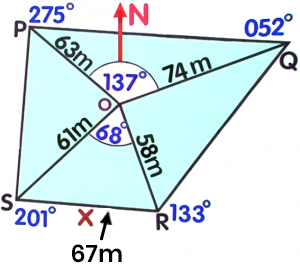 
`67m`