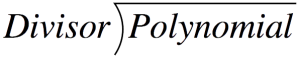Remainder Theorem
Try VividMath Premium to unlock full access
Time limit: 0
Quiz summary
0 of 4 questions completed
Questions:
- 1
- 2
- 3
- 4
Information
–
You have already completed the quiz before. Hence you can not start it again.
Quiz is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Loading...
- 1
- 2
- 3
- 4
- Answered
- Review
-
Question 1 of 4
1. Question
Write “F” if the binomial is a Factor and “N” if it is not a Factor of the polynomial:`P(x)=x^3+2x^2-5x-6`-
`(a) (x-2)=` (F, f)`(b) (2x-1)=` (N, n)`(c) (x+3)=` (F, f)
Hint
Help VideoCorrect
Correct!
Incorrect
Remainder Theorem
If `(P(x))/(x-a)` then `P(a)=r`where `r` is the RemainderNote that if `r=0`, then `(x-a)` is a Factor of `P(x)``(a)` Identify if `(x-2)` is a factor of `P(x)=x^3+2x^2-5x-6`.First, label the variable `a` by equating the binomial to `0` and solving for `x``x-2` `=` `0` `x-2` `+2` `=` `0` `+2` Add `2` to both sides `x` `=` `2` Hence, `a=2`Next, substitute `a` into the polynomial to solve for `r``P(x)=x^3+2x^2-5x-6``a=2``P(a)` `=` `r` Remainder Theorem `P(a)` `=` `a^3+2a^2-5a-6` Replace `x` with `a` `P(2)` `=` `2^3+2(2)^2-5(2)-6` Substitute `a=2` `=` `8+2(4)-10-6` `=` `8+8-10-6` `=` `16-16` `r` `=` `0` Since `r=0`, we can say that `(x-2)` is a factor of `P(x)=x^3+2x^2-5x-6``(b)` Identify if `(2x-1)` is a factor of `P(x)=x^3+2x^2-5x-6`.First, label the variable `a` by equating the binomial to `0` and solving for `x``2x-1` `=` `0` `2x-1` `+1` `=` `0` `+1` Add `1` to both sides `2x` `=` `1` `2x``-:2` `=` `1``-:2` Divide both sides by `2` `x` `=` `1/2` Hence, `a=1/2`Next, substitute `a` into the polynomial to solve for `r``P(x)=x^3+2x^2-5x-6``a=1/2``P(a)` `=` `r` Remainder Theorem `P(a)` `=` `a^3+2a^2-5a-6` Replace `x` with `a` `P(1/2)` `=` `(1/2)^3+2(1/2)^2-5(1/2)-6` Substitute `a=1/2` `=` `1/8+2(1/4)-5/2-6` `=` `1/8+4/8-20/8-48/8` `r` `=` `(-63)/8` Since $$r\neq0$$, we can say that $$(2x-1)$$ is not a factor of $$P(x)=x^3+2x^2-5x-6$$`(c)` Identify if `(x+3)` is a factor of `P(x)=x^3+2x^2-5x-6`.First, label the variable `a` by equating the binomial to `0` and solving for `x``x+3` `=` `0` `x+3` `-3` `=` `0` `-3` Subtract `3` from both sides `x` `=` `-3` Hence, `a=-3`Next, substitute `a` into the polynomial to solve for `r``P(x)=x^3+2x^2-5x-6``a=-3``P(a)` `=` `r` Remainder Theorem `P(a)` `=` `a^3+2a^2-5a-6` Replace `x` with `a` `P(-3)` `=` `(-3)^3+2(-3)^2-5(-3)-6` Substitute `a=-3` `=` `-27+2(9)+15-6` `=` `-27+18+15-6` `=` `-33+33` `r` `=` `0` Since `r=0`, we can say that `(x+3)` is a factor of `P(x)=x^3+2x^2-5x-6``(a)` Factor`(b)` Not a factor`(c)` Factor -
-
Question 2 of 4
2. Question
Solve for the remainder of:`(8x+x^2+3)divide(3+x)`- `r=` (-12)
Hint
Help VideoCorrect
Well Done!
Incorrect
Remainder Theorem
If `(P(x))/(x-a)` then `P(a)=r`where `r` is the RemainderLong Division
Use long division when a polynomial is divided by a binomialNote that if `r=0`, then `(x-a)` is a Factor of `P(x)`Method OneFind the remainder using long divisionFirst, arrange the terms in descending order of powers.$$\mathsf{P}$$(Polynomial) `=` `8x+x^2+3` `=` `x^2+8x+3` $$\mathsf{Divisor}$$ `=` `3+x` `=` `x+3` < `=` 
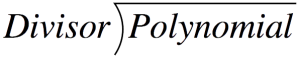Next, solve for each term of the quotientFirst term of the quotient:Divide the first term of the Polynomial by the first term of the Divisor. Place this above the Polynomial`x^2dividex` `=` `x` 
Multiply `x` to the divisor. Place this under the Polynomial`x``(x+3)` `=` `x^2+3x` 
Subtract `x^2+3x` and write the difference one line below
Drop down `3` and repeat the process to get the second term of the quotient
Second term of the quotient:Divide the first term of the bottom expression by the first term of the Divisor. Place this above the Polynomial`5xdividex` `=` `5` 
Multiply `5` to the divisor. Place this under the Polynomial`5``(x+3)` `=` `5x+15` 
Subtract `5x+15` and write the difference one line below
`-12` is not divisible by the divisor (`x+3`) anymore, hence `r=-12``r=-12`Method TwoFind the remainder using remainder theoremFirst, label the variable `a` by equating the divisor to `0` and solving for `x``x+3` `=` `0` `x+3` `-3` `=` `0` `-3` Subtract `3` from both sides `x` `=` `-3` Hence, `a=-3`Next, substitute `a` into the polynomial to solve for `r``P(x)=8x+x^2+3``a=-3``P(a)` `=` `r` Remainder Theorem `P(a)` `=` `8a+a^2+3` Replace `x` with `a` `P(-3)` `=` `8(-3)+(-3)^2+3` Substitute `a=-3` `=` `-24+9+3` `r` `=` `-12` `r=-12` -
Question 3 of 4
3. Question
Solve for the remainder of:`(x^4-3x^3+2x+8)/(x-2)`- `r=` (4)
Hint
Help VideoCorrect
Excellent!
Incorrect
Remainder Theorem
If `(P(x))/(x-a)` then `P(a)=r`where `r` is the RemainderLong Division
Use long division when a polynomial is divided by a binomialNote that if `r=0`, then `(x-a)` is a Factor of `P(x)`Method OneFind the remainder using long divisionFirst, notice that the term with the power of `2` is missing. Add this term to the polynomial before using long division$$\mathsf{P}$$(Polynomial) `=` `x^4-3x^3+2x+8` `=` `x^4-3x^3+``0x^2``+2x+8` $$\mathsf{Divisor}$$ `=` `x-2` `=` 
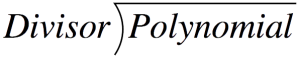Next, solve for each term of the quotientFirst term of the quotient:Divide the first term of the Polynomial by the first term of the Divisor. Place this above the Polynomial`x^4dividex` `=` `x^3` 
Multiply `x^3` to the divisor. Place this under the Polynomial`x^3``(x-2)` `=` `x^4-2x^3` 
Subtract `x^4-2x^3` and write the difference one line below
Drop down `0x^2` and repeat the process to get the second term of the quotient
Second term of the quotient:Divide the first term of the bottom expression by the first term of the Divisor. Place this above the Polynomial`-x^3dividex` `=` `-x^2` 
Multiply `-x^2` to the divisor. Place this one line below`-x^2``(x-2)` `=` `-x^3+2x^2` 
Subtract `-x^3+2x^2` and write the difference one line below
Drop down `2x` and repeat the process to get the third term of the quotient
Third term of the quotient:Divide the first term of the bottom expression by the first term of the Divisor. Place this above the Polynomial`-2x^2dividex` `=` `-2x` 
Multiply `-2x` to the divisor. Place this under the Polynomial`-2x``(x-2)` `=` `-2x^2+4x` 
Subtract `-2x^2+4x` and write the difference one line below
Drop down `8` and repeat the process to get the fourth term of the quotient
Fourth term of the quotient:Divide the first term of the bottom expression by the first term of the Divisor. Place this above the Polynomial`-2xdividex` `=` `-2` 
Multiply `-2` to the divisor. Place this under the Polynomial`-2``(x-2)` `=` `-2x+4` 
Subtract `-2x+4` and write the difference one line below
`4` is not divisible by the divisor (`x-2`) anymore, hence `r=4``r=4`Method TwoFind the remainder using remainder theoremFirst, label the variable `a` by equating the divisor to `0` and solving for `x``x-2` `=` `0` `x-2` `+2` `=` `0` `+2` Add `2` to both sides `x` `=` `2` Hence, `a=2`Next, substitute `a` into the polynomial to solve for `r``P(x)=x^4-3x^3+2x+8``a=2``P(a)` `=` `r` Remainder Theorem `P(a)` `=` `a^4-3a^3+2a+8` Replace `x` with `a` `P(2)` `=` `2^4-3(2)^3+2(2)+8` Substitute `a=2` `=` `16-3(8)+4+8` `=` `16-24+4+8` `r` `=` `4` `r=4` -
Question 4 of 4
4. Question
Solve for the remainder of:`(a) (x^4-5x^3+3x^2+8)/(x-2)``(b) (3x^4-x^2+2x+6)/(x+1)`-
`(a) r=` (-4)`(b) r=` (6)
Hint
Help VideoCorrect
Great Work!
Incorrect
Remainder Theorem
If `(P(x))/(x-a)` then `P(a)=r`where `r` is the Remainder`(a)` Solve for the remainder of `(x^4-5x^3+3x^2+8)/(x-2)`.First, label the variable `a` by equating the divisor to `0` and solving for `x``x-2` `=` `0` `x-2` `+2` `=` `0` `+2` Add `2` to both sides `x` `=` `2` Hence, `a=2`Next, substitute `a` into the polynomial to solve for `r``P(x)=x^4-5x^3+3x^2+8``a=2``P(a)` `=` `r` Remainder Theorem `P(a)` `=` `a^4-5a^3+3a^2+8` Replace `x` with `a` `P(2)` `=` `(2)^4-5(2)^3+3(2)^2+8` Substitute `a=2` `=` `16-5(8)+3(4)+8` `=` `16-40+12+8` `r` `=` `-4` `(b)` Solve for the remainder of `(3x^4-x^2+2x+6)/(x+1)`.First, label the variable `a` by equating the divisor to `0` and solving for `x``x+1` `=` `0` `x+1` `-1` `=` `0` `-1` Subtract `1` from both sides `x` `=` `-1` Hence, `a=-1`Next, substitute `a` into the polynomial to solve for `r``P(x)=3x^4-x^2+2x+6``a=-1``P(a)` `=` `r` Remainder Theorem `P(a)` `=` `3a^4-a^2+2a+6` Replace `x` with `a` `P(-1)` `=` `3(-1)^4-(-1)^2+2(-1)+6` Substitute `a=-1` `=` `3(1)-1-2+6` `=` `3-1-2+6` `r` `=` `6` `(a)-4``(b) 6` -